Macroscopic and Microscopic Approaches | Concept of continuum
Macroscopic and Microscopic Approaches
The behaviour of matter can be studied by two approaches
1. Macroscopic approach, 2. Microscopic approach
The behaviour of matter can be studied by two approaches
1. Macroscopic approach, 2. Microscopic approach
Difference of sets Let A and B be two sets. The difference of A and B written as A – B, is the set of all those elements of A which do not belong to set B Thus A – B={ x : x ∈ A and x ∉ B} or A – B={ x…
Disjoint sets Two sets A and B are said to be disjoint, if A∩B=Φ. If A∩B≠Φ, then A and B are said to be intersecting or overlapping sets As shown in Fig(vi) Fig(vi) Example If A={ 1, 2, 3, 4, 5, 6 }, B={ 7, 8, 9, 10, 11 } and C= { 6, 8, 10,…
Conservation of Energy In the early part of 19th century the scientist developed the concept of energy and hypothesis that it can be neither created nor destroyed ; this come to be known as the law of the conservation of energy. The first law of thermodynamics is merely one statement of this…
Semi-Open or Semi-Closed interval If a and b are two real numbers such that a < b, then the sets (a, b] = { x : x ∈ R, a < x ≤ b} and [a, b)={ x : x ∈ R, a ≤ x < b are known as semi-open or semi-closed intervals ….
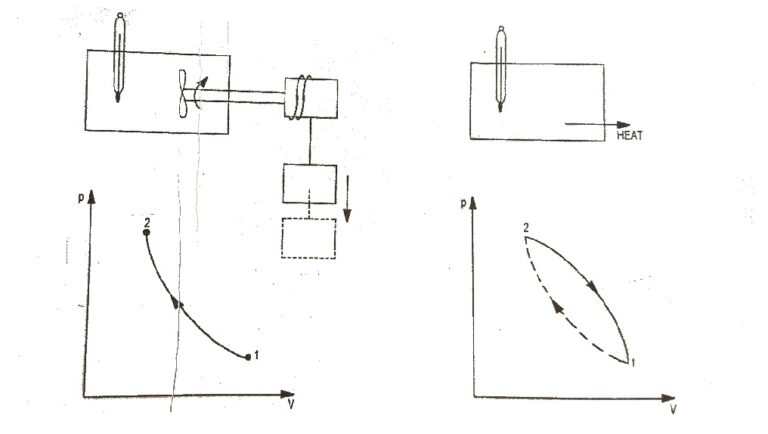
First Law of a Closed System Undergoing a Cycle : The first law of thermodynamics states that during any cycle a system undergoes the cyclic integral of heat is equal to the cyclic integral of the work. Fig.1 First Law of Thermodynamics To illustrates this, consider the gas as a system in…
Intersection of sets Let A and B be two sets. The intersection of A and B is the set of all those elements that belongs to both A and B. See in Fig(v) shaded region show A∩B We denote A intersection B by notation “A ∩ B” Thus A∩B = { x : x ∈…